MEDIAN – DEFINITION AND CALCULATION

MEDIAN – DEFINITION AND CALCULATION

Contents hide
1) MEDIAN - DEFINITION AND CALCULATION
2) INTRODUCTION
3) MEDIAN
4) CALCULATION
5) MERITS OF THE MEDIAN
6) DEMERITS OF THE MEDIAN

MEDIAN - DEFINITION AND CALCULATION

INTRODUCTION

Mean, median and mode all three are Central Tendency or Averages. The Arithmetic mean lies under Mathematical Average and the other two Median and Mode are types of Positional Averages.

A measure of central tendency is a typical value around which the figures congregate”. The value of central tendency or average always lies between the minimum and maximum values.

MEDIAN

  • Median is an average which divides a distribution into 2 equal halves.
  • When we arrange values in ascending order, the middle value is the median.
  • Median is represented by the letter ‘Md”.
  • Median can also be calculated by:
  • Ungrouped data
  • Discrete series
  • Continuous series

CALCULATION

1.Individual series or Ungrouped data

Step 1- Arrange the data in ascending or descending order.

Step 2- Apply the formula

MEDIAN - DEFINITION AND CALCULATION

Where,

Md = Median

N = total number of items= Sf

2. Discrete series

Step 1- Arrange the data in ascending or descending order

Step2-   Find the cumulative frequencies

Step 3- Apply formula

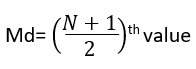
MEDIAN - DEFINITION AND CALCULATION

Where,

Md = Median

N = total number of Items

3. Continuous series :

Step 1- Find the cumulative frequencies

Step2- Find out the median class by using

Step 3- Apply the formula

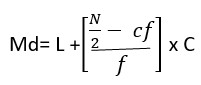
MEDIAN - DEFINITION AND CALCULATION

Where,

L = lower limit of the median class

cf = cumulative frequency prior to the median class

f = actual frequency of the median class

c = class interval of the median class.

MERITS OF THE MEDIAN

  • It is simple to calculate and easy to understand.
  • It can be located by inspection . So it is an inspectional value
  • The value of median is unaffected by extreme values
  • Its value generally lies in the distribution
  • It is further used in statistical calculations

DEMERITS OF THE MEDIAN

  • Median is not a familiar average like mean.
  • In the large ungrouped data, the calculation of median is tedious because of the rearranging data into ascending or descending order.
  • Since it is positional average , its value is not influenced by each and every observations.
  • The median value is affected by sampling fluctuations.

Leave a Reply

This Post Has 9 Comments

  4. DavidLam 21/01/2023 Reply

    Thanks Writing on this topic. this article is really useful.

  6. Quintanieasymn 14/12/2024 Reply

    Купить Haval – только у нас вы найдете цены ниже рынка. Быстрей всего сделать заказ на купить хавал джулион можно только у нас!
    хавал джулиан цена
    купить хавал джулиан – http://www.jolion-ufa1.ru

  7. Garmcrypto7nog 14/12/2024 Reply

    Blockchain technology news Unlock the potential of Blockchain technology with our comprehensive news coverage that keeps you informed about the latest trends, breakthroughs, and insights in the industry. Whether you are a seasoned expert or just starting out, our updates will ensure you never miss a beat.

    https://t.me/s/cryptonetlake

  8. DanielKatte 15/12/2024 Reply

    Greatest news for all us

  10. RonaldAmord 16/12/2024 Reply

    useful Website